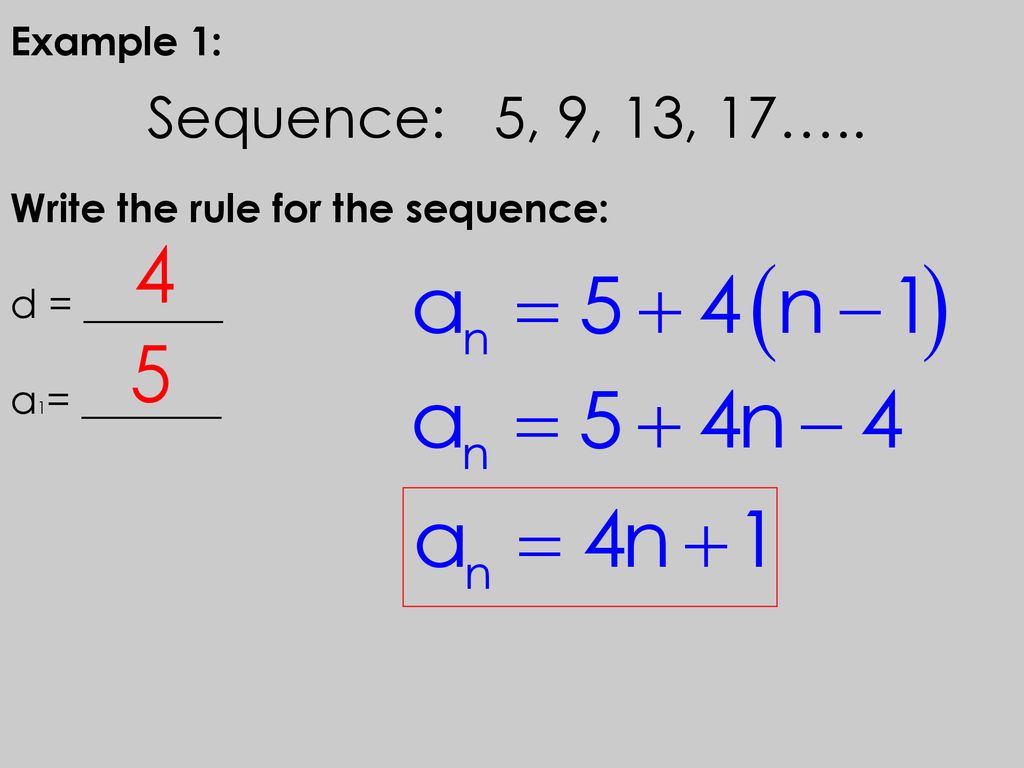
What is the rule of pattern 5 9 13 17
Answer and Explanation:
The sequence is 5 , 9 , 13 , 17 , ⋯ . As the difference between consecutive terms is equal, the sequence is an arithmetic progression. The first term of the AP is 5 and the common difference is 4. So, the general term of the AP is a n = 5 + 4 ( n − 1 ) .
What is the nth term rule of 5 9 13 and 17
This series is an AP because the difference between the two consecutive terms is the same. Hence, the nth term of 5,9,13,17,— is (4n+1).
What is the next number in the sequence a 5 9 13 17 21 25
29
So the next number in the sequence = 25 + 4 = 29.
What is the general term of the sequence 1 5 9 13
Answer and Explanation:
We will first check if this is an arithmetic sequence by seeing if there is a common difference between succeeding pairs of terms. Thus, the nth term rule for the given sequence is a n = 4 n − 3 .
How to find the nth term of the sequence of 5 9 13 17 and 21
nth term examplesFind the nth term for the sequence 5, 9, 13, 17, 21, …Here, 9 − 5 = 4.The common difference d = 4 .2 Multiply the values for n = 1, 2, 3, … by the common difference.Here, we generate the sequence 4n = 4, 8, 12, 16, 20, …. (the 4 times table).The nth term of this sequence is 4 n + 1 .
What is the common difference of the sequence 5 9 13 17 21
{1,5,9,13,17,21,25,…} is an arithmetic sequence with common difference of 4 .
Is 1 5 9 13 17 arithmetic or geometric
arithmetic sequence
{1,5,9,13,17,21,25,…} is an arithmetic sequence with common difference of 4 .
What is the sum of the 30 terms of the arithmetic sequence 5 9 13 17
The obtained sum is 1890.
Which of the following is the common difference in the arithmetic sequence 5 9 13 17
4
For example, in the arithmetic sequence 1, 5, 9, 13, 17, …, the difference is always 4. This is called the common difference. If the first term of the sequence is a and the common difference is d, then the arithmetic sequence can be written as a, a+d, a+2d, a+3d, …, a+(n−1)d, …
What is the sum of the first 30 terms of 5 9 13 17
The obtained sum is 1890.
What is the recursive rule for 1 5 9 13 17
The sequence starts out as 1, 5, 9, 13, 17, ___, … By inspection we can see that the common difference is 4, the first value is 1, n is 6. And that the next value is 21. By using the formula T(n)=a+(n-1)d, We can find the next value.
What is the common difference of the arithmetic sequence 5 9 13 17
4
And, we know that the difference between the consecutive numbers is 4. Hence, we have found the common difference of the arithmetic sequence 5, 9, 13, 17,…. The common difference is 4.
What type of sequence is 5 9 13 17 21
arithmetic sequence
1, 5, 9, 13, 17, 21, 25, 29, . . ., 4n+1, . . . In general, the terms of an arithmetic sequence with the first term a0 and common difference d, have the form an = dn+a0 (n=0,1,2,…).
What are the next three terms of the arithmetic sequence 5 9 13 17
5,9,13,17,21,25,29,33,37.
What are the first four terms of the arithmetic sequence 5 9 13 17
This means that, as n increases by 1, the result increases by 4. This means that there must be a 4n term in the formula for the sequence. So if we apply that, we get the result: 4, 8, 12, 16. This is clearly 1 out for each result, so we can deduce that the formula really is:nth term = 4n + 1.
What will be the sum of 23 terms of the sequence 5 9 13 17
Sn=n2[2a+(n−1)d]∴S23=232[2(5)+(23−1)4] =232×98 =1127.
What is the recursive rule for 2 3 5 8 13
And the most classic recursive formula is the Fibonacci sequence. The Fibonacci sequence is as follows: 0, 1, 1, 2, 3, 5, 8, 13, 21,… Notice that each number in the sequence is the sum of the two numbers that precede it. For example, 13 is the sum of 5 and 8 which are the two preceding terms.
What is the rule of recursive rule
We learned that a recursive rule is a rule that continually takes a previous number and changes it to get to a next number. For example, our counting numbers is a recursive rule because every number is the previous number plus 1.
What is the common difference of 5 9 13 17 21
4
{1,5,9,13,17,21,25,…} is an arithmetic sequence with common difference of 4 .
Which term of the arithmetic sequence 5 9 13 17 so on is 401
Answer: The 100th term in the sequence 5, 9, 13, 17,.. is term 401.
What is the common difference of the arithmetic sequence 5 9 13 17 21
4
Example 1:
{1,5,9,13,17,21,25,…} is an arithmetic sequence with common difference of 4 .
What rule is used in the sequence 5 9 13 17 21
This is an arithmetic sequence since there is a common difference between each term. In this case, adding 4 to the previous term in the sequence gives the next term. In other words, an=a1+d(n−1) a n = a 1 + d ( n – 1 ) . This is the formula of an arithmetic sequence.
What is the recursive rule for the sequence − 2.7 − 8.3 − 13.9 − 19.5 − 25.1 responses
Summary: The recursive rule for the sequence -2.7, -8.3, -13.9, -19.5, -25.1,… is adding -5.6.
What is the sequence of 5 7 9 11
Arithmetic Progressions
An arithmetic progression is a sequence where each term is a certain number larger than the previous term. The terms in the sequence are said to increase by a common difference, d. For example: 3, 5, 7, 9, 11, is an arithmetic progression where d = 2. The nth term of this sequence is 2n + 1 .
What is the recursive rule for 3 7 11 15
This is an arithmetic sequence since there is a common difference between each term. In this case, adding 4 to the previous term in the sequence gives the next term. In other words, an=a1+d(n−1) a n = a 1 + d ( n – 1 ) .


